Introduction to the Integration by Substitution Method

In calculus, the reverse of the derivative of a function is known as integration. There are different methods to solve integrals. One of these is the substitution method. This method is used to solve integrals with an easy method. Let’s understand more about the substitution method and the types involved in this method. 

Integration by Substitution

The integration is a process of finding antiderivatives of a function. It is a method of adding or finding sum of all parts to calculate area or volume of whole part. Integration by substitution is a method of solving integrals. This method involves the substitution of another function or variable in order to find the solution by using integration techniques.

The method of substitution allows us to evaluate complex integrals in a more easy way. We can use different types of substitutions depending on the integrand. For this, the method of substitution is further divided into two types. These are namely, 

1. U-substitution Method
2. Trigonometric Substitution

Let’s discuss both types of the substitution methods and their calculations. 

U-Substitution Method

 The u-substitution method is a type of substitution method. In this method, we use a parameter ‘u’ as a substitution parameter which makes the given integrand easier to manage. Let’s understand how to solve integrals by using the u-substitution method calculator in the following example.

Example of U-substitution method

Consider that we need to solve the integral of sin2x/1+cos^2x. As the function contains a trigonometric function. But it seems a little bit tricky because of the angle involved. The integral of sin2x/1+cos^2x can be written as;

$I=\int \frac{\sin 2x}{1+\cos^2x}dx$

Since we know that,

$\sin 2x=2\sin x\cos x$

Therefore assume that

$u = \cos x\quad\text{and}\quad du =-\sin x dx$

Since we are using a parameter u to rewrite the given integral, therefore,this method is known as u-substitution. Using this substitution in the integral,

$I = ∫\frac{-2u}{1+u^2}.du$

Since

$\frac{d}{dx}(1+u^2) = 2u$

Now integrating with respect to u we get,

$I = -\ln|1+u^2| + c$

Now substituting the value of u here,

$I = -\ln|1+\cos^2x|+c$

Hence we have calculated the integral of sin 2x by 1+cos^2x by using the u-substitution method. You can also use our u-substitution calculator to avoid long-term calculations.

Trigonometric Substitution Method

It is another method of calculating the integration by using the substitution method. In this method, we use trigonometric functions for substitution. This method is used to solve integrals with nonlinear functions. We use the following substitution to evaluate integration by using this method.

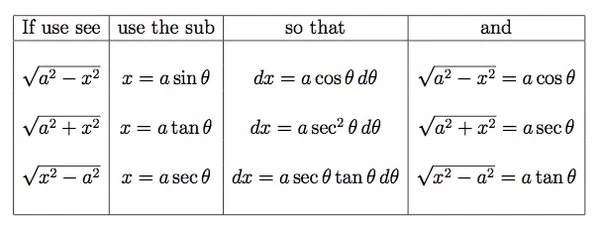
Let’s solve the following example by using trigonometric substitution.

Example of Trigonometric Substitution 

Consider that we want to solve the integral of sqrt(1-x^2) which is written as;

$I=\int\sqrt{1-x^2}dx$

In the trigonometric substitution, we substitute the variable with a trigonometric function. Now suppose that,

$x=\sin \theta$

and

$dx=\cos \theta d\theta$

Since,

$\cos \theta =\sqrt{1-\sin^2\theta}=\sqrt{1-x^2}$

Using the new substitution in the above integral.

$I=\int \cos \theta .\cos\theta d\theta$

We can write it as;

$I=\int \cos^2\theta d\theta $$

By using the following trigonometric formula,

$\cos^2 \theta=\frac{1+\cos 2\theta}{2}$

The integral will become, 

$I=\int \frac{1+\cos 2\theta}{2}d\theta $

Separating the integrals,

$I=\int \frac{1}{2}d\theta +\int \frac{\cos 2\theta}{2}d\theta $

Integrating, each term,

$I=\frac{\theta}{2}+\frac{\sin 2\theta}{4}=\frac{\theta}{2}+\frac{2\sin \theta \cos \theta}{4}$ 

$I=\frac{\theta}{2}+\frac{\sin \theta\cos \theta}{2}$

Writing cos in terms of sin .

$I=\frac{\theta}{2}+\frac{1}{2}\times \sin \theta\sqrt{1-\sin^2\theta}$

Now substituting the value of theta,

$I=\frac{1}{2}\sin^{-1}x+\frac{1}{2}x\sqrt{1-x^2}+c$

Hence we have calculated the integral of sqrt(1-x2). You can also use our trig-substitution calculator to evaluate integrals by using trigonometric substitution.

How do you evaluate integrals by using integration by substitution method?

The method of integration by substitution involves two different methods i.e. u-substitution and trigonometric substitution. Here we provide you a step-by-step method to evaluate integrals by using this method. Use the following steps.

1. Identify the type of integrand. If it is a combination of two functions, we will use the method of u-substitution. 
2. If the function is a nonlinear function with a square root, we will use the method of trigonometric substitution.
3. After choosing the type of substitution method, use the integration techniques to simplify the integrals.
4. After solving, substitute the solution back to the original function. 

Use our integration by parts calculator that provides a step-by-step solution of the integrals.

Conclusion

The method of integration by substitution is a technique of solving integrals by using two types of substitutions i.e, u-substitution and the trigonometric substitution. These types provide an easy way to manage complex integrals. By understanding this method, we can solve many complex problems in calculus.