Introduction of Area under the curve calculator
The area under curve calculator is an online tool which is used to calculate the definite integrals between the two points. This calculator will help in finding the definite integrals as well as indefinite integrals and gives the answer in a series of steps.
It helps in solving the equations and gives results with accurate answers. The definite integrals or indefinite integrals can be easily calculated by using this online calculator. The use of this calculator will save your time and energy that you spent in solving the long divisions or equations manually. It is also free of cost and easy to use.
How to find the Area Under Curve Calculator online?
The area under the curve calculator is known as the most advanced online calculator which can easily be searched with the help of the internet to solve integral online. This advanced online tool can be searched or found by simple steps. These simple and easy steps are:
- On the internet, Google will help in finding the curve integral calculator. Primarily, you can type the main keyword of this calculator Area Under the curve Calculator on the search bar of Google. Google will direct you to the main page of the calculator immediately. It's all on up to you to choose the correct option and select the main calculator.
- Google will show you various results after typing the area under a curve calculator. The main thing while choosing this calculator is to understand the major instructions of the calculator and guidelines about the use of this calculator. Select the online calculator carefully according to your need.
- The most common method is to find the integration calculators website and search this calculator directly from here. The calculator integral has developed many of the integration calculators from which you can easily find this calculator. All these calculators are free & accurate.
Related: Also Find u substitution calculator or trigonometric substitution integral calculator with steps on google for evaluating integration by substitution methods.
Benefits of using Area under the Curve Calculator
We know that finding the area under the curve is a lengthy or long procedure to solve manually. Therefore, the use of this calculator has provide different benefits which can be mentioned as follows:
- The area under curves calculator will help in calculating the problems or equations in just a few seconds and solve the functions step by step.
- This area under the curve calculator with steps helps in saving your time and keeps you away from manual calculations.
- It helps in practicing the concepts of area under the curve online and you can also learn about it by using this calculator.
- This calculator provides a plot and possible intermediate steps of long divisions method online or equations and its possible number of steps.
- It also provides the real part, imaginary part and alternate form of the definite integrals or indefinite integrals within the result.
- This calculator is free of cost and helps in calculating the accurate results.
To calculate lengthy and complex integral, use our partial fraction integral calculator & integration by parts calculator with steps.
Results provided by Integration Area Under Curve Calculator
The results which are obtained by the area under the curve calculator are very accurate. It gives the answers to every function step by step which can be easily understood. The results attained by this calculator are definitive and have simple steps to understand this process properly. It will help in finding the real part, imaginary part, intermediate steps, alternate form of the integrals and series expansion of the integrals within the results.
Is the Area Under Curves Calculator reliable?
The results provided by area under curve calculator with steps are reliable. It helps in calculating the functions more quickly and provides accurate results step by step. It helps in consuming the time and solving the problems in just a few seconds. This calculator will give the chances of learning the area under the curve process and provide the accurate results which can easily be understood.
How to use the Area Under the Curve Integration Calculator?
The use of an area under the curve calculator will ease you to solve the functions or equations. It has simple instructions to follow. Few of the basic steps for using this calculator are:
- The first step is to enter the function from the calculator or load the example.
- Select whether you want to evaluate the area under the curve functions as per definite integral or indefinite integral.
- If you want to select a definite integral, then select the upper bound and lower bound for the process of integration and if you want to select the indefinite integrals then there is no need of selecting the upper bound or lower bound. It automatically disappears after selecting the indefinite integral.
- You can also select the variables with respect to x, y, z according to your function.
- Then click on the “Calculate” button to process the function. This calculator will give the results in a few seconds and give you the solution step by step.
There are many other useful calculators which will be beneficial for you while studying calculus. Just like you can determine riemann sum calculator or trapezoidal rule calculator for calculating area under the curve in different ways.
Why to use the Curve Integral Calculator?
The area under a curve calculator is known as the best source of calculating the definite integrals the two points, integration, functions, expressions and definite integrals or indefinite integrals. It helps in finding the area under the curve process.
The basic motive of using this calculator is to attain the best results in just a short time. It helps in selecting the variables and definite integrals as well as indefinite integrals as per your calculation choice. It helps in calculating the area under the curve, free of cost and provides simple results in a series of steps.
Thus we hoped you enjoyed the one of the most advanced and demanded tool of integrals. Integral calculator provides a numerous of different tools that can help in the integration. One may also try convergent or divergent integral calculator that help us to calculate improper integral of a funcion.
Visual form of your input is also shown below the input line that help user to analyze his integrand function under the integral. So keep enjoying while calculating the integral with us!
FAQ's
What is Area Under Curve?
The area of under the curve is the area between the curve and its coordinates. It is calculated by the help of infinite and definite integrals. The process of integration is mostly used to find the area under the curve, if its equation and the boundaries are known. It is denoted as;
$A\;=\;∫_a^bf(x)dx{2}$
If you have values infinite and definite integrals, then you can find the area under the curve calculator with steps online.
You can also use area between the curves calculator for more specific step by step results with graph and plot.
What is the Area Under the Normal Curve if the Z-Score given is 2.14?
To find the area under the normal curve with z-score, the z-score table for normal distribution is used. So by using the table, the area with z-score 2.14 is 0.01618. The area under curve calculator also provides the accurate results.
When is Area Under the Curve Negative?
The area under the curve can be negative after the process of integration. The negative sign with area indicates that the area of the curve lies below the x-axis is larger. It just indicates that area is below the x-axis. If you want to find area under the graph online, you can use area under graph calculator.
How to find Area Under Curve using Integration?
It is easy to find the area under the curve by using the technique of integrals if the boundaries of the curve is known. You can find area under the curve online by using integral area calculator. For example to find the area under the curve f(x)=〖3x〗^2+2 from x=1 to x=2, it will be written as,
$A=∫_1^2(3x^2)dx$ $A=|\frac{3x^3}{3}|_1^2\;=\;\frac{3(2)^3}{3}\;-\;\frac{3(1)^3}{3}$ $A=8-1=7{2}$
What is the Curve Integral?
An integral that is evaluated along a curve is known as curve integral. There are different terms used for it. For example, curve integral, curvilinear integral and path integral are used sometimes. It is known as curve integral because it helps to find the area under a curve by integration.
Can we calculate area between two curves using area under the graph calculator?
Area between & under curve are two different things. Area between curve is the measure of the region occupied by the space between the two curves. While, area under curve is the measure of the region occupied by the curve.
You can estimate area under curve using rectangles calculator and calculate area under the curve and get steps. We also have area between two curves calculator with steps so that you can calculate the region occupied by the space between the two curves.









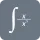








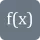

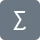







 Google Play
Google Play
 App Store
App Store

0 Comment