Introduction to the Surface Integral Calculator
In calculus, an integral calculator is a fundamental tool that is used to calculate areas, volumes, and their generalizations. When it comes to solving complex problems in physics, engineering, or computer graphics, the surface integral is an essential mathematical concept. However, manually calculating surface integrals can be time-consuming and prone to errors. That's where the surface integral calculator comes in handy. It's a powerful tool that simplifies and automates the process, making accurate calculations much easier.
Formula Used by Surface Integral Calculator with steps
To understand how the surface integral solver works, it's essential to grasp the underlying formula it employs. The general formula for calculating a surface integral over a parametric surface S is as follows:
$∫∫_S F · dS = ∫∫_D F(r(u,v)) · (r_u × r_v) dA$
Where F represents the vector field, S is the parametric surface, D denotes the parameter domain, r(u,v) defines the position vector of S, (ru × rv) is the cross product of the partial derivatives, and dA represents the area element.
How Does the Surface Integral Calculator Work?
This calculator simplifies the calculation process by automating the formula mentioned earlier. It employs advanced algorithms and mathematical techniques to efficiently perform surface integral calculations. By entering the appropriate input values, such as the vector field and the parametric surface, the calculator swiftly computes the result, saving time and minimizing the risk of manual errors. Similar to the surface integral calculator, you can also use our line integral calculator which provides you with a step-by-step accurate solution.
How to Use the Surface Integral Calculator with Steps?
Using the surface integral finder is straightforward and user-friendly. Let's explore the step-by-step process:
- Input the vector field: Provide the function or formula representing the vector field you wish to evaluate.
- Specify the parametric surface: Define the parametric equations that describe the surface over which the integral will be computed.
- Determine the parameter domain: Identify the range of values for the parameters (u and v) that define the surface.
- Select the appropriate coordinate system: Decide whether to use Cartesian, cylindrical, or spherical coordinates, depending on the nature of the problem.
- Enter the values: Input the necessary values into the calculator, such as the vector field components, the parametric equations, and the parameter domain.
- Calculate the surface integral: Click the "Calculate" button, and the Surface Integral Calculator will perform the computations, providing you with the result.
By following these simple steps, the calculator enables you to quickly obtain accurate surface integral values, facilitating your mathematical analyses and problem-solving. Also try our definite integral calculator to evaluate integrals with limits.
Why Use a 2D Surface Integral Calculator?
The surface integral of a sphere calculator offers a specialized solution for evaluating surface integrals over two-dimensional surfaces. In various scenarios, working with 2D surfaces is more practical or relevant, and this calculator streamlines the process specifically for such cases. It allows you to input the necessary parameters and swiftly calculates the surface integral, providing you with precise results.
Benefits of Using a Surface Integral of a Sphere Calculator
The Surface Integral Calculator is a versatile tool, and one of its noteworthy applications is the computation of surface integrals over spheres. Here are some benefits of utilizing this calculator:
- Time-saving: Manually calculating surface integrals over spheres can be time-consuming, especially for complex problems. The calculator accelerates the process, providing results in seconds.
- Accuracy: The calculator eliminates the potential for human error, ensuring accurate and reliable computations. This is particularly crucial when dealing with intricate mathematical concepts.
- Versatility: The calculator allows you to evaluate surface integrals over spheres of varying radii and positions, accommodating a wide range of mathematical scenarios.
How to Find a Surface Integral Online Calculator?
Finding a reliable and user-friendly calculator online is essential for efficient mathematical calculations. Numerous websites and online platforms offer this tool, allowing you to access it with ease. A simple internet search with relevant keywords like "Surface Integral Calculator" will provide you with a list of options. Be sure to select a reputable website or a trusted mathematical resource to ensure the accuracy and reliability of the calculator.
In conclusion, the surface integral calculator is an indispensable tool for accurately computing surface integrals. By automating the complex mathematical calculations involved, this calculator saves time, minimizes errors, and enhances efficiency in various fields of study. Whether you're a student, researcher, or professional, utilizing the Surface Integral Calculator empowers you to solve intricate problems and gain valuable insights.
Related: You Can Also Find Vector Field Calculator and Parametric Integral Calculator For More Information.
Frequently Asked Questions
What is the surface integral?
Surface integral is the generalization of multiple integrals to integrate over surfaces. It is also known as the generalization of double integral. It integrates a curve from one dimension into 2 dimensions.
How do you solve surface integrals?
To solve the surface integral, the integrand is integrated two times over the given interval. Different integration techniques are used to find surface integral. The formula to calculate surface integral is:
$∫∫_S F · dS = ∫∫_D F(r(u,v)) · (r_u × r_v) dA$
Is the Surface Integral Calculator accurate?
Yes, the Surface Integral Calculator employs advanced algorithms and mathematical techniques to ensure accurate and reliable calculations.



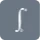






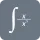
















 Google Play
Google Play
 App Store
App Store

0 Comment