Introduction to Arc Length of Polar Curve Calculator
The polar arc length calculator is an online tool that calculates the arc length of a polar curve. It helps you to find the distance between two points on the curve line in polar coordinates. You need to enter the circle's angle and radius as input.
In geometry, the arc length is crucial because it is one of the applications of the integrals. Since this concept involves integrals, it takes work to compute. We introduce you to an online tool to avoid this difficulty so that you can calculate the arc length of a circle in polar coordinates.
Formula used by Arc Length in Polar Coordinates Calculator
In geometry, the arc length of a circle in polar coordinates is the distance between two points on the curved line of a circle. As the integral provides a way to calculate the area under the curve, it also helps calculate the circle's arc length in polar coordinates. The formula of the polar arc length calculator is:
$ L =∫^b_a \sqrt{1+(f'(x))^2} {2}$
Where f’(x) is referred to as the circle's radius, the definite integral is used to calculate the arc length of a polar curve because it is impossible to calculate it by using any other geometric formula. The above formula is used by the polar curve integral calculator to assist you in doing calculations of arc length.
How to use the Polar Curve Length Calculator?
Every tool works when you provide its input. Therefore, you must provide input values such as radius and central angle to use arc length of entire polar curve calculator. There are some simple steps to use this tool. These are:
- In the first step, you need to enter the central angle of the circle. In this step, you have to enter the circle's angle value to calculate the arc length of a polar curve.
- Now, enter the radius of the circle.
- Review the input values and click on the calculate button.
After clicking the calculate button, the arc length polar curve calculator will provide you arc length for the given values within a few moments. You can also calculate the area between two curves by using our area between curves calculator.
Why to use the Polar Curve Arc Length Calculator Online?
The polar curve intersection calculator is one of our best online integral tools. It allows you to do calculations without any external help. It also helps you to understand the calculations or results step-by-step. Therefore, using arc length of polar curve calculator allows you to find the arc length of a polar curve quickly.
In geometry, many concepts may be confusing and tough to evaluate. It is because you must remember some formulas to learn these concepts thoroughly. But the length of the polar curve calculator assists you in doing calculations without remembering any formula. Therefore, it would be best to use this tool.
Benefits of using Arc Length of Polar Coordinates Calculator
There are many benefits that you can get by using our arc length of polar function curve calculator. Also, these are helpful in learning and understanding maths. Some of them are listed below.
- It saves you from doing tricky long, and manual calculations.
- It is easy to use because you need to perform some easy and simple steps.
- Arc length of polar curve calculator helps the students to solve many real-life problems related to geometry.
- It is a free online tool; you don’t need to pay any fee.
- It executes faster and gives accurate results.
How to find the arc length of entire polar curve calculator?
The polar curve arc length calculator is our advanced and multi-featured online mathematical tool. You can easily find it online or follow the given steps.
- Use the relevant keywords to search from your desired search engine.
- You will receive different results from your search engine. Choose the result relevant to the calculator from the given results.
- A list of related tools will be provided on the website page of the calculator.
- Choose the arc length of the polar graph calculator from the list. You could also use one of our many tools, like the indefinite integral solver.
FAQ's
How do you find the arc length of a polar curve?
The arc length of a polar curve can be calculated by using its formula. It includes a definite integral to calculate arc length. The formula is:
$ L =∫^b_a \sqrt{1+(f'(x))^2} {2}$
What do you mean by polar curve?
A shape constructed by using a polar coordinate system is called a polar curve. Points on polar curves have varying distances from the origin, depending on the angle taken off the positive x-axis to measure distance.









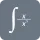



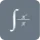





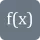

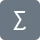







 Google Play
Google Play
 App Store
App Store

0 Comment