Introduction to Improper integral calculator
A improper integral calculator is used to help the mathematicians and students in measuring the improper integral. This improper integral calculator with steps easily finds the improper integral by using techniques of integration with in seconds. You just have to put equation and put the lower bound to get the answer. You will have to select one lower bound as other one will be infinity.
The improper integral convergence calculator helps to determine whether your inserted function is divergent or convergent. If you are a calculus student or a mathematician then this improper integral convergence calculator is a perfect supporter for you.
Because it will be handy in completing assignments and if you are struct in your question. This is a daily use tool to calculate improper integral.
For daily life problem solving, we also recommend to use our other tools such as multiple integral calculator with steps, integral triple calculator and many more which this integral calculator provides.
What is an improper integral calculator?
This is a tool used to evaluate improper Integral Calculator which works to provide the integrated value for the improper integral. The improper definite integral calculator is well manufactured to assist the users in computing complex improper integral functions in the blink of an eye. The results will be accurate due to the awesome algorithm working in its backend.
As when we study calculus, the type of integration that gives the area between the curve is an improper integral. It is necessary to determine the upper and lower limit of such integrals. We can classify improper integrals as definite integrals.
Related: To calculate definite integrals and indefinite integrals use definite integral online calculator and integral indefinite calculator for free easily.
However, the calculation regarding improper integrals is complex and we bring a simpler method to perform such calculation. If you want to know this method stick to the description below for the complete procedure.
The improper integral calculator does not provide you the substitution methods of integration. Thus, for this purpose you need to use u substitution calculator or trigonometric substitution integral calculator.
Formula used in Improper Integration
Improper integrals formula is as follows
$\int_0^∞f(x)dx{2}$
To understand this formula it is neccessary to know that one of limit must be infinite. As if any limit which may be a or b is infinite than it will be called as improper integration.
This improper integral calculator with steps solves the whole proces in its algorithm to give answer in seconds with steps using this formula.
How to use the improper integral calculator
Many of you may be keen to understand the actual process to get started with this improper integral calculator. Here are some simple steps which you need to follow to get the best results in no time. however, some essentials need to be kept in mind while using our improper integral calculator online.
Step 1: write the function inside the "enter function" box. You can also load examples to try the calculator. There are many different types of examples incorporated inside this amazing calculator.
Step 2: Choose the desired variables from the list containing X, Y, and Z variables.
Step 3: limits are quite essential here to clearly define the function. You must add lower and upper bound limits before calculation.
Step 4: In the final step you just have to click on the “calculate” button to get quick results. This tool also determines whether the function converges or not.
Related: Calculate integrals through disc method easily by using our volume disk method calculator and also find volume using washer method calculator respectively.
FAQ's
What is the improper integral of 1/x^2?
Integral will be $-x\frac13+c$ As you will have to add a coefficient to to solve otherwise integral will not converge
Related: We also provide some unique calculator to complete your need of integrals such as laplace transformation calculator and fourier sine transform calculator
How to find improper integrals online?
If you are looking for a quick and easier way to find out improper integral, then you just need to search for our Improper integral calculator. It gives you the best possible results with reliability. You can also follow the above-mentioned steps to compute improper integrals.
However, you must have a pure understanding of the concept to avoid any misconceptions. It is necessary to determine the exact limits to finding improper integrals online.
We hope you have liked our tool. Also try our other tools to make your work easy as integral calculator provides many online itegration tools for your ease. You can try our some of the famous calculators such as cylindrical shells volume calculator and integration by partial fraction calculator.
Is improper integral convergent?
An improper integral can be convergent and divergent depending on the associated limit. It converges when the corresponding limit exist and is a finite number, we say that the improper integral converges.
When is improper integral convergent and divergent?
The convergence and divergence of improper integrals depends on the limit associated with the definite integrals as:
$ \int_a^∞ f(x) \;=\; \lim \limits_{t \to ∞} \int_a^t f(x) dx {2}$
If the limit exists and takes a finite number after the integration then we say that the improper integral is convergent. But if the limit does not exists, the improper integral is said to be divergent.
We can calculate both forms by using convergent or divergent integral calculator above.
Is 1/x an improper integral?
The function 1/x can be written in the form of improper integral.
$ \int_a^∞ \frac{1}{x} dx \;=\; \lim \limits_{t \to ∞} \int_a^t \frac{1}{x} dx {2}$
So it is improper integral. But it is divergent because the limit does not exist.
What are improper integrals?
The concept of improper integrals is an extension of definite integrals. We can define improper integral as, if f is continuous over the interval then the improper integral is:
$ \int_a^∞ f(x) \;=\; \lim \limits_{t \to ∞} \int_a^t f(x) dx {2}$
Where f is integrated from a to t.
Is gamma function improper integral?
The gamma function is an important property of improper integrals which is defined as for x>0,
$ Γ(x) \;=\; \int_0^∞ t^{x-1} e^{-t} dt {2}$
So it is obvious that gamma function is an improper integral.









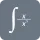
















 Google Play
Google Play
 App Store
App Store

0 Comment