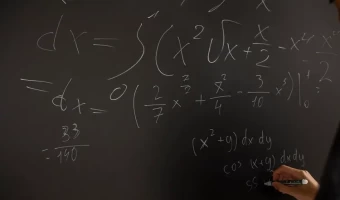There are different ways through which we can evaluate the indefinite integral of cos(x) - 1/x as an infinite series. Two of the main representation series are:

1. Power series representation of cos(x)
2. Geometric series representation of 1/x

Power series representation of cos(x)

The power series representation of cos(x) is given by:

$\cos(x) = 1 - \frac{x^2}{2!} + \frac{x^4}{4!} - \frac{x^6}{6!} +\cdots $

Geometric series representation of 1/x

The geometric series representation of 1/x is given by:

$\frac{1}{x} = 1 + (-1)x + (-1)^2x^2 + (-1)^3x^3 + \cdots $

Substituting these series into the integral, we get:

$\int (\cos(x) - \frac{1}{x}) dx$ $= \int (1 - \frac{x^2}{2!} + \frac{x^4}{4!} - \frac{x^6}{6!} + ... - 1 - (-1)x - (-1)^2x^2 - (-1)^3x^3 - ...) dx$

$= \int (1 - \frac{x^2}{2!} - (-1)x + \frac{x^4}{4!} - (-1)^2x^2 - ...) dx$

$= x - \frac{x^3}{3!} - (-1) \frac{x^2}{2} + \frac{x^5}{5!} + ...$

This series can be further simplified to:

$x - \frac{x^3}{6} + \frac{x^5}{120} + \dotsb $

Therefore, the indefinite integral of cos(x) - 1/x as an infinite series is:

$x - \frac{x^3}{6} + \frac{x^5}{120} + \dotsb + C$ where C is the constant of integration.